本文共 9967 字,大约阅读时间需要 33 分钟。
文章目录
1 概述
Logback是在java社区广泛使用的日志框架之一,它是Log4j的替代品。Logback提供了比Log4j更快的实现,更多可选择的配置,以及归档log文件更大的灵活性。
2 Logback的架构
包括三个类:Logger,Appender和Layout。
- Logger, 是log信息的上下文,是跟应用交互以生成log信息之用;
- Appender,负责日志输出的组件;
- Layout,用来格式化日志信息等。
3 例子
这里创建一个springboot工程,添加以下依赖
3.1 Maven依赖
org.slf4j slf4j-api 1.7.30 ch.qos.logback logback-core 1.2.3 ch.qos.logback logback-classic 1.2.3
3.2 基本的配置和代码demo
在resources下面创建logback.xml文件,添加以下配置:
%d{HH:mm:ss.SSS} [%thread] %-5level %logger{36} - %msg%n
创建一个Example:
@Slf4jpublic class Example { public static void main(String[] args) { log.info("Example log from {}", Example.class.getSimpleName()); }} 运行得到以下结果:
09:59:36.226 [main] INFO com.logback.demo.Example - Example log from Example。4 Logger context
@Slf4j是来自lombok的注解,相当于如下代码:
private static final Logger log = LoggerFactory.getLogger(Example.class);
可以从编译后的class文件,反编译后看到。
4.1 logger继承结构
Logger context的继承类似于java的继承。
- 所有的logger都是root logger的后代;
- 如果一个logger没有指定level,那么它的level将继承上一个祖先logger.
- root logger的默认level是DEBUG。
注意:日志级别分为5种,从小到大依次是TRACE, DEBUG, INFO, WARN 和ERROR。低于指定级别的日志将不打印,例如我们指定日志级别是INFO,那么低于INFO的TRACE和DEBUG都不打印。
上代码:
public class LoggerHierarchyExample { @Test public void testLoggerHierarchy() { // 祖先logger,未指定级别,它将继承root logger的级别,root logger默认debug // SLF4J's的抽象logger是没有实现setLevel方法,这里是用ch.qos.logback.classic.Logger Logger ancestorLogger = (Logger) LoggerFactory.getLogger("com"); // 父logger,指定级别为info,不继承祖先logger的级别 Logger parentLogger = (Logger) LoggerFactory.getLogger("com.logback"); parentLogger.setLevel(Level.INFO); // 子logger,未指定级别,继承最近的父logger的日志级别,为info。 Logger childLogger = (Logger) LoggerFactory.getLogger("com.logback.demo"); ancestorLogger.debug("This message is logged because debug == debug"); ancestorLogger.trace("This message is not logged because trace < debug"); parentLogger.warn("This message is logged because WARN > INFO."); parentLogger.debug("This message is not logged because DEBUG < INFO."); childLogger.info("INFO == INFO"); childLogger.debug("DEBUG < INFO"); } @Test public void testRootLogger() { // 没有指定level,继承root logger的level为debug Logger logger = (Logger) LoggerFactory.getLogger("com.logback"); logger.debug("Hi there!"); // root logger,重新设定level为error Logger rootLogger = (Logger) LoggerFactory.getLogger(org.slf4j.Logger.ROOT_LOGGER_NAME); logger.debug("This message is logged because DEBUG == DEBUG."); rootLogger.setLevel(Level.ERROR); logger.warn("This message is not logged because WARN < ERROR."); logger.error("This is logged."); }} testLoggerHierarchy()结果为:
10:49:16.713 [main] DEBUG com - This message is logged because debug == debug10:49:16.715 [main] WARN com.logback - This message is logged because WARN > INFO.10:49:16.715 [main] INFO com.logback.demo - INFO == INFO
testRootLogger() 结果为:
10:50:06.087 [main] DEBUG com.logback - Hi there!10:50:06.089 [main] DEBUG com.logback - This message is logged because DEBUG == DEBUG.10:50:06.089 [main] ERROR com.logback - This is logged.
4.2 参数化打印日志
实际编码中,看到很多如下日志打印方式:
log.debug("Current count is " + count); 这种方式的弊端:即使日志打印level设定为info,都会执行字符串的拼接,无疑会白白的损耗性能。
以下方式也不可取,虽然不执行拼接,但仍然做了一次判断。
if(log.isDebugEnabled()) { log.debug("Current count is " + count);} 更好的方式是使用占位符的方式 , 避免字符串拼接,避免日志level的判断。
log.debug("Current count is {}" , count); 占位符{}允许接收任何object,并且调用其toString方法来记录日志。
@Slf4jpublic class ParameterizedExample { @Test public void testParameterizedExample() { String message = "This is a String"; Integer zero = 0; User user = new User().setId("123").setName("Tim"); try { log.debug("Logging message: {}", message); log.debug("Going to divide {} by {}", 42, zero); log.debug("user is {}", user); int result = 42 / zero; } catch (Exception e) { log.error("Error dividing {} by {} ", 42, zero, e); } }} @Data@Accessors(chain = true)public class User { private String id; private String name;} 5 详细配置
logback默认行为:假如它没有找到任何配置文件,它将默认创建一个ConsoleAppender,并且关联到root logger。
5.1 logback查找配置文件顺序
- logback按照顺序在classpath中查找配置文件:logback-test.xml, logback.groovy, logback.xml ;
- 假如上面的file都没有找到,则启动Java的 查找com.qos.logback.classic.spi.Configurator的实现;
- 假如以上都没有,则启动logback默认的行为: 创建一个ConsoleAppender,并且关联到root logger。
5.2 基本配置解释
所有的配置都在里面。
%d{HH:mm:ss.SSS} [%thread] %-5level %logger{36} - %msg%n
这里配置了一个Appender,类型是ConsoleAppender,名称是STDOUT。
标签是root logger,level是debug,关联到STDOUT的Appender。
5.3 解决日志故障配置
logback的配置文件可以很复杂,因此logback提供内置机制来排查故障。为查看logback自身的日志,可以打开debug模式:
打印出的日志如下所示:
15:17:24,550 |-INFO in ch.qos.logback.classic.LoggerContext[default] - Could NOT find resource [logback-test.xml]15:17:24,550 |-INFO in ch.qos.logback.classic.LoggerContext[default] - Could NOT find resource [logback.groovy]15:17:24,550 |-INFO in ch.qos.logback.classic.LoggerContext[default] - Found resource [logback.xml] at [file:/E:/10_git/LogbackDemo/target/classes/logback.xml]15:17:24,699 |-INFO in ch.qos.logback.core.joran.action.AppenderAction - About to instantiate appender of type [ch.qos.logback.core.ConsoleAppender]15:17:24,703 |-INFO in ch.qos.logback.core.joran.action.AppenderAction - Naming appender as [STDOUT]15:17:24,733 |-INFO in ch.qos.logback.core.joran.action.NestedComplexPropertyIA - Assuming default type [ch.qos.logback.classic.encoder.PatternLayoutEncoder] for [encoder] property15:17:24,775 |-INFO in ch.qos.logback.classic.joran.action.RootLoggerAction - Setting level of ROOT logger to DEBUG15:17:24,775 |-INFO in ch.qos.logback.core.joran.action.AppenderRefAction - Attaching appender named [STDOUT] to Logger[ROOT]15:17:24,776 |-INFO in ch.qos.logback.classic.joran.action.ConfigurationAction - End of configuration.15:17:24,778 |-INFO in ch.qos.logback.classic.joran.JoranConfigurator@5606c0b - Registering current configuration as safe fallback point15:17:24.782 [main] INFO com.logback.demo.Example - Example log from ExampleDisconnected from the target VM, address: '127.0.0.1:50977', transport: 'socket'Process finished with exit code 0
5.4 自动重载配置
scan=true,表示打开自动扫描配置,默认为false。scanPeriod="15 seconds"表示每15秒扫描一次,也可以设置milliseconds, seconds, minutes, or hour,如果没设置,默认为1分钟。
5.5 修改Loggers
我们可以给任何logger配置level,如下配置:
%d{HH:mm:ss.SSS} [%thread] %-5level %logger{36} - %msg%n
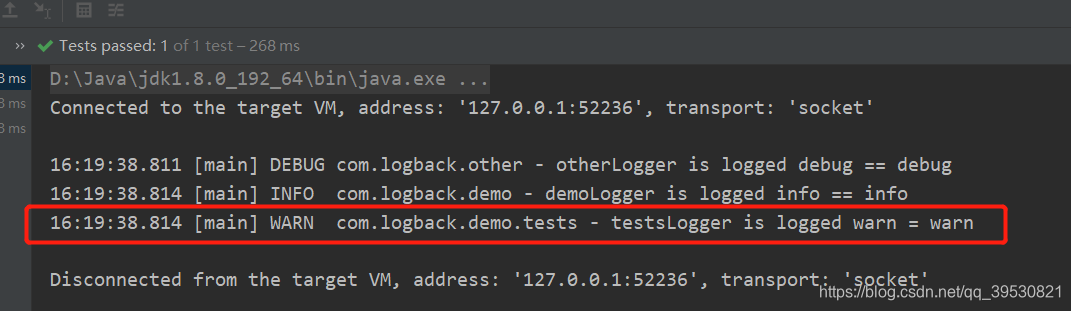
public class ModifyingLoggersExample { @Test public void testModify() { Logger otherLogger = LoggerFactory.getLogger("com.logback.other"); Logger demoLogger = LoggerFactory.getLogger("com.logback.demo"); Logger testsLogger = LoggerFactory.getLogger("com.logback.demo.tests"); otherLogger.debug("otherLogger is logged debug == debug"); demoLogger.debug("demoLogger is not logged debug < info"); demoLogger.info("demoLogger is logged info == info"); testsLogger.info("testsLogger is not logged warn > info"); testsLogger.warn("testsLogger is logged warn = warn"); }} 结果:
15:52:17.359 [main] DEBUG com.logback.other - otherLogger is logged debug == debug15:52:17.361 [main] INFO com.logback.demo - demoLogger is logged info == info15:52:17.362 [main] WARN com.logback.demo.tests - testsLogger is logged warn = warn
Logger同样能继承root logger的appender-ref。
5.6 变量替代
${LOG_DIR}/tests.log true %-4relative [%thread] %-5level %logger{35} - %msg%n
我们声明一个 名称是LOG_DIR, 值是/var/log/application,logback会将它的值注入到${LOG_DIR}。
6 Appenders
logback支持不进支持log以文件形式输出,还支持其他的形式。
6.1 ConsoleAppender
控制台输出,利用System.out 或 System.err输出。
6.2 FileAppender
FileAppender是把log输出到文件。
%d{HH:mm:ss.SSS} [%thread] %-5level %logger{36} - %msg%n tests.log true %-4relative [%thread] %-5level %logger{35} - %msg%n
运行ModifyingLoggersExample的testModify可以看到工程中多了一个文件tests.log.
我们可以同时在tests.log文件和控制台看到同样的输出信息;
 这是因为logger继承了来自 的<appender-ref , 如果要阻止,只需如下配置:添加additivity=“false”
这是因为logger继承了来自 的<appender-ref , 如果要阻止,只需如下配置:添加additivity=“false” 6.3 RollingFileAppender
有时候我们不需要将log信息一直输出到同个文件,而是根据时间,文件大小或者两者,将历史文件打包输出。
${LOG_DIR}/${LOG_FILE}.log ${LOG_DIR}/${LOG_FILE}.%d{yyyy-MM-dd}.gz 30 3GB %-4relative [%thread] %-5level %logger{35} - %msg%n
这里定义了文件路径和文件名,使用基于时间的TimeBasedRollingPolicy,不仅定义文件名,而且定义了每天打包一次文件。也可以按月打包一次:
${LOG_DIR}/%d{yyyy/MM}/${LOG_FILE}.gz
6.4 自定义Appender
参考这里:https://www.baeldung.com/custom-logback-appender。
7 Layouts
Layouts用于格式化log信息。也可以自定义Layout,但通常我们使用默认的PatternLayout 。
%d{HH:mm:ss.SSS} [%thread] %-5level %logger{36} - %msg%n
- %d{HH:mm:ss.SSS},表示小时,分钟,秒,毫秒;
- [%thread] ,表示线程;
- %-5level,表示日志level;
- %logger{36},表示logger的名,截取35个字符;
- %msg%n,%msg日志信息,%n表示换行。 可以参考:http://logback.qos.ch/manual/layouts.html#conversionWord
8 总结
我们学习了Logback的基本功能,了解了三个组件:Logger,Appender和Layouts,比较详细讲解了主要使用的FileAppender和RollingFileAppender.
代码地址:https://github.com/tobebetter9527/LogbackDemo。转载地址:http://qshwz.baihongyu.com/